TANGENTS I

The word tangent comes from the Latin word ‘tangens’ that means ‘to touch’, so it is the straight line that “just touches” the curve at that point.  The point where the tangent line and the curve meet is called the point of tangency

 

1. TANGENT LINE TO A CIRCLE ON ONE OF ITS POINTS.

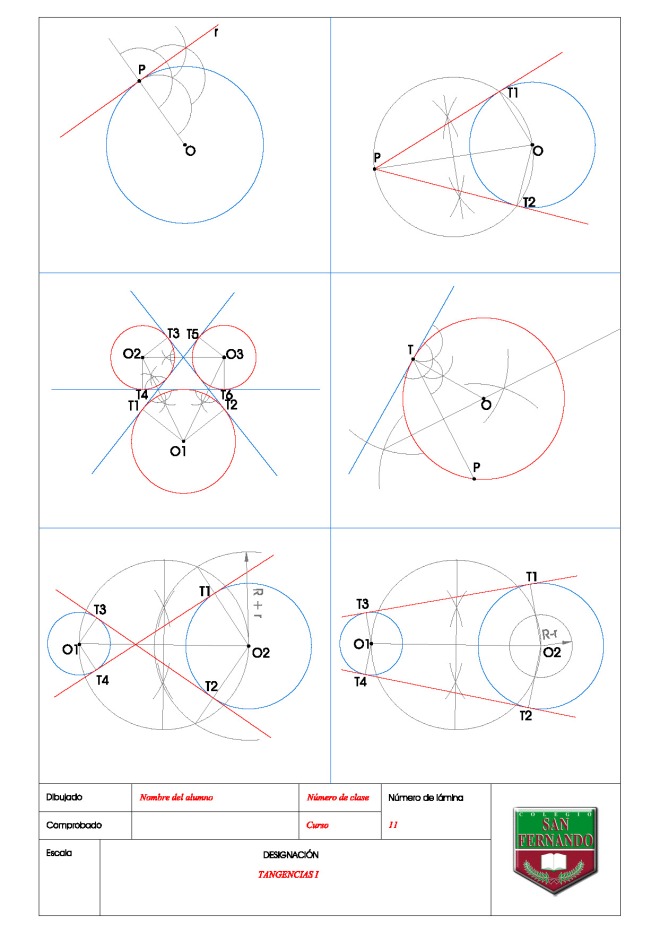
A line tangent to a circle on one of its points must be perpendicular to the radius of that circle on that point.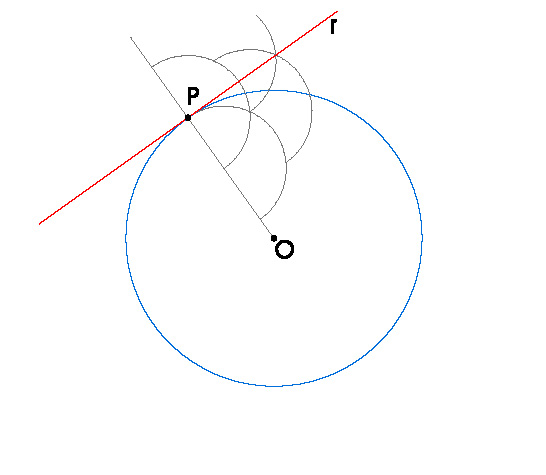
STEPS:

1. Draw the circle on wich we will draw the tangent. Label its centre with letter O.
2. Choose any point P on the circle.
3. Draw the radious OP.
4. Draw a perpendicular line to segment OP on its endpoint P.
5. The line “r” will be the perpendicular we were looking for.

2. TANGENT LINES TO A CIRCLE THAT PASSES THROUGH AN EXTERIOR POINT OF THAT CIRCLE.

When we start getting the tangents to a circle from an exterior point P, we can see that this problem has two solutions. To solve the exercise we must bear in mind two previous ideas:

• The perpendicular line to a circle on one of its points must be perpendicular to the radius of the circle on that point, so the triangles POT1 and POT2 are both right triangles.
• ‘Arco capaz’ concept for a particular angle, which is the locus of all the points from where the segment is seen at a given angle.

Here we need to see the segment OP at an angle of 90º, for getting that we will draw the circle which diameter is segment OP ( the same of having a central angle of 180º) so, all the inscribed angles that we draw from this central angle will have half of its measure, it is 90º. Arco capaz of a 90º angle

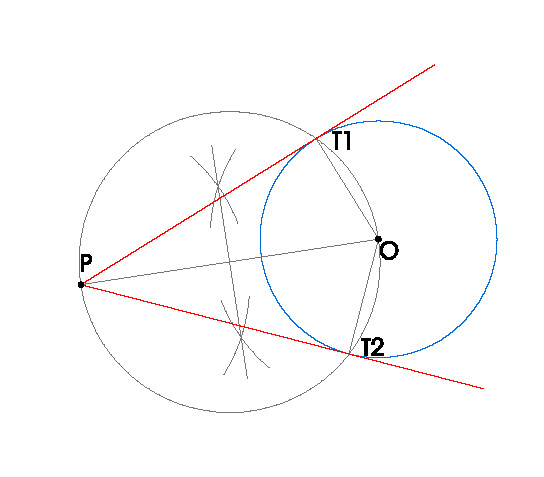
1. Draw a circle and label its centre with letter O.
2. Choose any point P, outside the cicle; this will be the point from where we will draw the tangent lines to the circle.
3. Join O and P.
4. Get the line bisector of segment OP.
5. Centring your compass on midpoint of segment OP, M, draw the circle that passes trough points O and P.
6. Where that circle crosses the data circle we will get the tangency points T1 and T2.
7. If we join point P with points T1 and T2, we will get the solutions of the exercise, lines t1 and t2.

3. TANGENT CIRCLES  TO THREE LINES THAT CROSS EACH OTHER.

The solution circles are the three excenter circles to the triangle that is form by the intersection of the three lines. The excenter are the intersection points of the angle bisectors of the exterior angles of the triangle. This is the third Apolonio’s problem, the problem itself has as a solution also the incircle of the triangle. To solve this problem we must remember angle bisector concept.The angle bisector is the locus of all the points which are at the same distance to the sides of an angle, therefore the angle bisector is the locus of al the circle centres of the circles that are tangent to both sides of the angle, as we had studied when we solve the Incenter problem.

4. TANGENT CIRCLE TO A GIVEN LINE ON A POINT OF IT “T” AND THAT PASSES THROUGH ANOTHER GIVEN POINT “P”.

To solve this problem we must remember line bisector concept, which is the locus of all the points on the plane that are at the same distance to the endpoints of a segment, therefore it is the locus of all the circle centres of the circles that passes through both endpoints of the segment.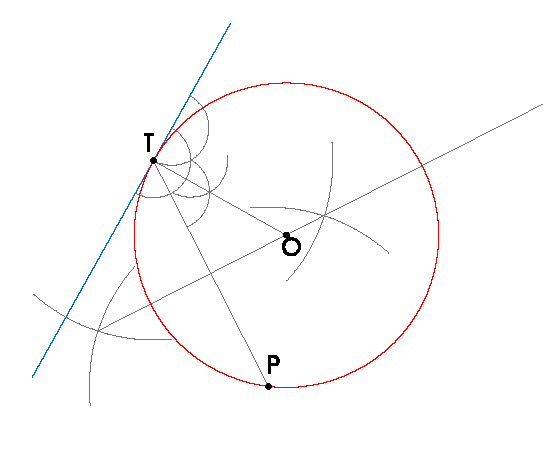

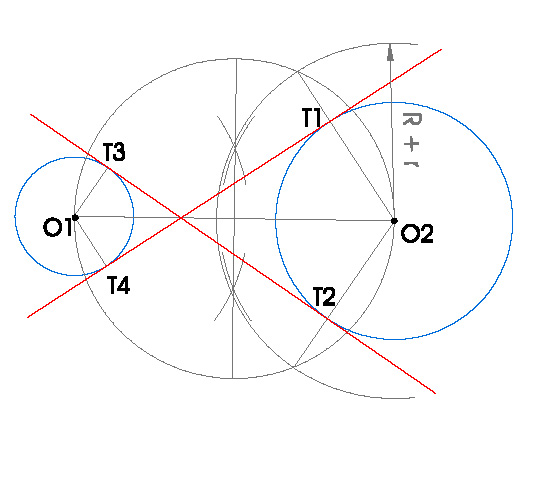
5. INNER TANGENT LINES TO TWO CIRCLES.

A new circle of radius r_{2 +} r₁ is drawn centered on O₂

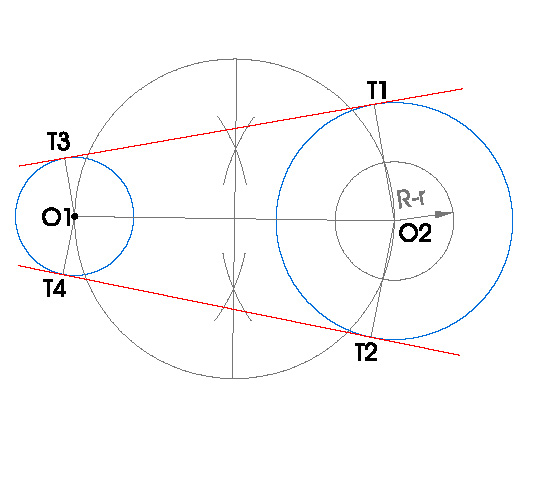
6. OUTER TANGENT LINES TO TWO CIRCLES.

A new circle of radius r_{2 –} r₁ is drawn centered on O₂

Solution of the complete drawing sheet: